
Understanding Fractions: Parts of a Whole
Fractions are fundamental in understanding how we represent parts of a whole. They play a crucial role in STEM education, helping learners of all ages develop their mathematical thinking and problem-solving skills. This page delves into the concept of fractions, offering engaging insights and practical applications that will inspire curiosity and foster critical thinking.
Fractions are a way to represent parts of a whole. Imagine you have a delicious pizza. If you want to share it with your friends, you need to divide it into equal parts. Each part of the pizza can be written as a fraction.
What is a Fraction?
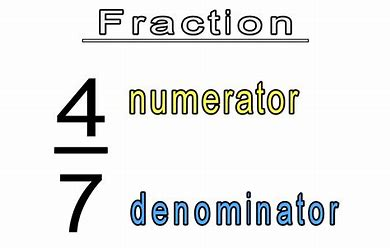
A fraction is made up of two numbers: the numerator and the denominator. The numerator tells you how many parts you have, and the denominator tells you how many parts the whole is divided into.
For example, if you divide a pizza into 4 equal parts and eat one slice, you’ve eaten 1/4 (one-fourth) of the pizza. Here, 1 is the numerator (how many parts you have), and 4 is the denominator (how many equal parts the pizza is divided into).
(The picture above, the pizza is divided into 4 equal parts. If you eat one slice, you have eaten 1/4 of the pizza.)
Why are Fractions Important?
Fractions help us understand parts of a whole. Whether you’re sharing food, dividing a cake, or measuring ingredients for a recipe, fractions help us know exactly how much we have.
Simple Fractions You Should Know:
- 1/2 (one-half): If you divide something into two equal parts, each part is 1/2.
- 1/3 (one-third): If you divide something into three equal parts, each part is 1/3.
- 1/4 (one-fourth): If you divide something into four equal parts, each part is 1/4.
These simple fractions will help you in many real-life situations, like cutting a pie or dividing a group of toys with your friends!
References:
- National Council of Teachers of Mathematics. (2014). "Understanding Fractions."
- Math Is Fun. (n.d.). "Fractions." Retrieved from https://www.mathsisfun.com/fractions.html.

What Are Fractions?
Fractions are a way to express numbers that are not whole. They consist of two parts: the numerator and the denominator. The numerator indicates how many parts we have, while the denominator shows how many equal parts make up a whole. For example, if you have a pizza sliced into eight equal pieces and you take three, you have 3/8 of the pizza. Understanding fractions is essential because they allow us to perform various operations, such as addition, subtraction, multiplication, and division, with numbers that do not divide evenly. This knowledge forms a foundation for more advanced mathematical concepts and real-world applications, such as cooking, budgeting, and understanding proportions in science experiments. By mastering fractions, students gain valuable skills that apply across multiple disciplines and everyday life.
The Importance of Visualizing Fractions
Visualizing fractions can significantly enhance comprehension and retention. Utilizing models, such as fraction circles or bars, can help learners grasp the concept of parts of a whole more intuitively. For instance, if we represent the fraction 1/2 with a circle divided into two equal parts, shading one part illustrates that one out of the two sections is being referred to. This method of visualization ties directly into hands-on STEM projects that encourage students to manipulate physical objects, thereby deepening their understanding. Additionally, exploring fractions through real-world scenarios, such as sharing food or measuring ingredients, makes the learning experience relatable and engaging. By integrating visual aids and practical applications, learners can develop a more profound appreciation for the significance of fractions in their daily lives and studies.

Hands-On Activities to Reinforce Fraction Concepts
STEM Scholars Hub emphasizes the importance of hands-on learning experiences to reinforce mathematical concepts, including fractions. Engaging in activities such as cooking, crafting, or building can provide practical opportunities to apply fraction knowledge. For instance, when baking, measuring ingredients can involve fractions—using 1/4 cup of sugar or 3/2 cups of flour. By collaborating with peers in community-based projects, students can tackle challenges that require fractional reasoning, fostering teamwork and critical thinking. Moreover, personalized guidance from Mr. Barbado ensures that each learner receives tailored support, whether they are beginners or advanced students. This inclusive approach guarantees that everyone, regardless of their starting point, can grasp and enjoy the fascinating world of fractions, making math not just a subject, but an exciting journey of discovery.
Ready to Explore Fractions?
Utilize the printable materials below.
Introduction to Fractions Lesson Plan.pdf